 NZMRI Summer School 2015
NZMRI Summer School 2015

Algebra, Number Theory and Discrete Geometry
Nelson, South Island, NZ
Speakers
Registration is now closed
Click here to see the list of people who registered.Kalman Summer Scholarships
Five students have received a Kalman Scholarship to attend the event. The scholarship is funded by the "Margaret and John Kalman Charitable Trust." The recipients of the scholarships are Matthew Conder, Shishay Gebregiyorgis, Lennox Leary, Thomas Robertson and Barak Shani.Venue
All lectures will be in the Tahuna beach holiday park conference centre. Very close to Nelson airport.Accommodation
We have reserved a number of rooms at the holiday camp and nearby motels. These include rooms suitable for individuals or couples, and also a few rooms suitable for families. We will be able to pay for the accommodation of students. However, due to a short budget, participating academics will be asked to contribute towards their accommodation costs. Accommodation will be given on a first-come first-serve basis. As Nelson is a busy place in the Summer, we recommend you register as soon as possible.Food
Morning teas and lunches will be provided for all participants, as will a BBQ dinner on Thursday night (guests of participants are welcome to join at cost). There is a selection of restaurants 10 minutes walk from the holiday park. The restaurants include Thai, German, gourmet pizza, fast food (McDonalds, KFC, fish and chips, Hell Pizza) and pub dining. Small local stores contain basic food items. Large supermarkets (and all other shopping) can be found in nearby Nelson (5-6km from Tahunanui).Schedule
The conference will begin in the morning of Monday 12 January and will finish on Friday 16 at noon. Afternoons will be free as usual.Conference registration will start around 5pm on Sunday. There will be a reception with finger food from 6pm till 8pm. Wednesday will be free all day and evening.
| Monday 12/1 | Tuesday 13/1 | Wednesday 14/1 | Thursday 15/1 | Friday 16/1 | |
| 9am | Deligne | Lehrer | Schoof | Weiss | |
| 10am | Praeger | Deligne | Lehrer | Schoof | |
| 11am | Tea/Coffee | Tea/Coffee | Tea/Coffee | Tea/Coffee | |
| 11:30am | Weiss | Praeger | Deligne | Lehrer | |
| 12:30pm | Lunch | Lunch | Lunch | Lunch | |
| 5pm | Schoof | Weiss | Praeger | ||
| 6pm | Public lecture (TBA) | ||||
| 6:30pm | BBQ till 9pm |
Program
Professor Deligne talked on What are modular forms of half-integral weight ?Abstract : Modular forms of integral weight can be viewed as sections of a line bundle over a moduli space (or stack) of elliptic curves. That this makes sense over Z explains rationality and integrality properties of their Fourier expansions. Similar properties hold in the half-integral case. We will explain how it can be given a similar understanding. It involves treating on an equal footing square roots of the Lie algebra of an elliptic curve, and "double coverings" of its Tate module.
Notes in french are available here.
Professor Lehrer talked on Fundamental theorems of invariant theory.
Abstract is available here. Slides are available here.
Professor Praeger talked on Simple group factorisations and applications in combinatorics.
Abstract : We will look at factorisations G=AB, and how this can be interpreted to describe symmetries of mathematical systems, e.g. whether one has the full symmetry group or not; whether a system is a "Cayley object"; whether one can embed a graph in a Riemann surface. Other kinds of factorisations, such as G=ABA, describe flag-transitive incidence structures.
Slides are available here, here and here.
Professor Schoof talked on Curves over finite fields.
Abstract : The lectures will be about curves over finite fields with many rational points. There are many problems in this area with applications in error-correcting codes and cryptography. Stepanov's proof of the Riemann hypothesis for curves over finite fields will be presented. Applications of class field theory to the construction of curves over finite fields with many rational points will be given. Algorithms to count points on elliptic curves over finite fields will be explained.
Notes are available here.
Professor Weiss talked on An Introduction to Buildings.
Abstract : We will give an introduction to Tits' theory of buildings. We will start with Coxeter groups and then define buildings as edge colored graphs satisfying two simple axioms. We will present some examples, make a few comments about the origin of the notion of a building, describe the basic properties of arbitrary buildings and give an overview of some of Tits' classification results. Time permitting, we will say something about Moufang sets, Tits indices and the fixed points of a group acting on a building. The only prerequisite for these lectures is some exposure to the basic notions of group theory.
Slides are available here.
Contact persons - organising commitee
A/Prof. Steven Galbraith, University of Auckland (s.galbraith@auckland.ac.nz)A/Prof. Dimitri Leemans, University of Auckland (d.leemans@auckland.ac.nz)
About the speakers
Pierre Deligne

|
Pierre Deligne is considered (one of) the best living mathematician(s) in the world. He has won all prestigious prizes in mathematics: Fields Medal in 1978, Crafoord prize in 1988, Balzan prize in 2004, Wolf prize in 2008 and Abel prize in 2013.
He is known for his work in algebraic geometry and number theory. He pursues a fundamental understanding of the basic objects of arithmetical algebraic geometry - motive, L-functions, Shimura varieties - and applies the methods of algebraic geometry to trigonometrical sums, linear differential equations and their monodromy, representations of finite groups, and quantization deformation. His research includes work on Hilbert's twenty-first problem, Hodge theory, the relations between modular forms, Galois representations and L series, the theory of moduli, tannakian categories, and configurations of hyperplanes.
Pierre Deligne is Professor Emeritus at the Institute for Advanced Study in Princeton. He is an honorary member of the Moscow Mathematical Society and of the London Mathematical Society. He is a foreign honorary member of the American Academy of Arts and Sciences and a member of the American Philosophical Society. He is also a foreign member of the Royal Swedish Academy of Sciences. |
Gus Lehrer

| Gustav Lehrer is a leading expert in representation theory, algebraic geometry and algebra. He is a professor at the University of Sydney where he spent most of his career. He is particularly known for developing with Bob Howlett a branch of representation theory now called the Howlett-Lehrer theory and for cellular algebras which he invented with his former student John Graham. His very considerable research contributions led to his election to the Australian Academy of Science in 1998. |
Cheryl Praeger
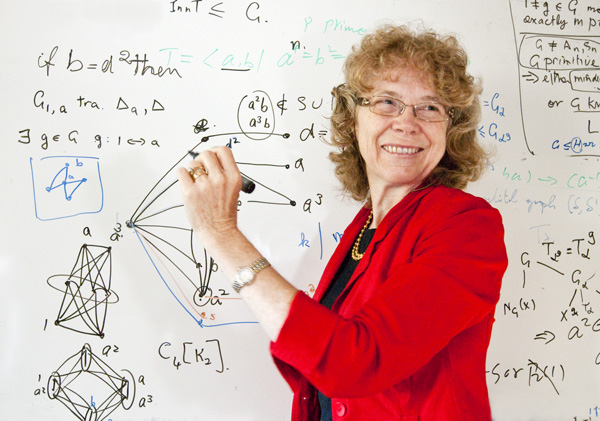
| Cheryl Praeger is a leading research mathematician in the areas of Group Theory and Combinatorics. Her research exploits the finite simple group classification to solve group theoretic, combinatorial, geometric, and computational problems. She has published more than 340 research articles and four research monographs, and is within the top 300 most highly cited mathematicians world-wide. Cheryl Praeger is recipient of the 2013 Thomas Ranken Lyle Medal and the 2011 Moyal Medal. She is the first woman President of the Australian Mathematical Society, a Member of the Order of Australia, a Fellow of the Australian Academy of Science, the first Pure Mathematician awarded an Australian Research Council Federation Fellowship, and the first Australian-based mathematician on the Executive Committee of the International Mathematical Union. She provides outstanding support and promotion of mathematics in Australia and internationally. |
René Schoof

| René Schoof received his PhD from the University of Amsterdam. He is a professor at Università di Roma "Tor Vergata" in Rome, Italy. His research interests include Algebraic Number Theory, Arakelov Theory, Iwasawa Theory, Computational Number Theory and Abelian Varieties. He invented the first polynomial-time algorithm for counting points on elliptic curves over finite fields, which has major applications in number theory and cryptography. He wrote a book on Mihailescu's proof of the Catalan's conjecture. |
Richard Weiss

| Richard Weiss is a leading expert in the theory of buildings. Buildings are geometric structures discovered and studied over a lifetime by the great Jacques Tits at the Collège de France. Richard Weiss has written four books about buildings over the last twenty years and is currently at work on one more. The first, which he wrote together with Tits, gives an extension of Tits' earlier theory of spherical buildings. In the second, a monograph called "The Structure of Spherical Buildings", he revisits this earlier theory, and in the third, he presents a new algebraic theory of certain exceptional buildings. The fourth, entitled "The Structure of Affine Buildings", contains a reworking of the classification results of Bruhat and Tits for affine buildings. Richard Weiss was awarded a Humboldt Research Prize in 2004 and a Mercator Professorship in 2012. He is the William Walker Professor of Mathematics at Tufts University and an honorary professor at the University of Birmingham. |